Math

Card Table Proof of the Pythagorean Theorem

Posted by Dave Richeson on

We own a standard card table that we leave tucked away in the basement until the kids want to have a lemonade stand on the front sidewalk or we need the extra table space for a large Thanksgiving dinner. It is the standard kind with legs that fold underneath it so it is easy to store.

Interestingly, when the legs are folded, the underside of the table encodes a famous proof of the Pythagorean theorem.

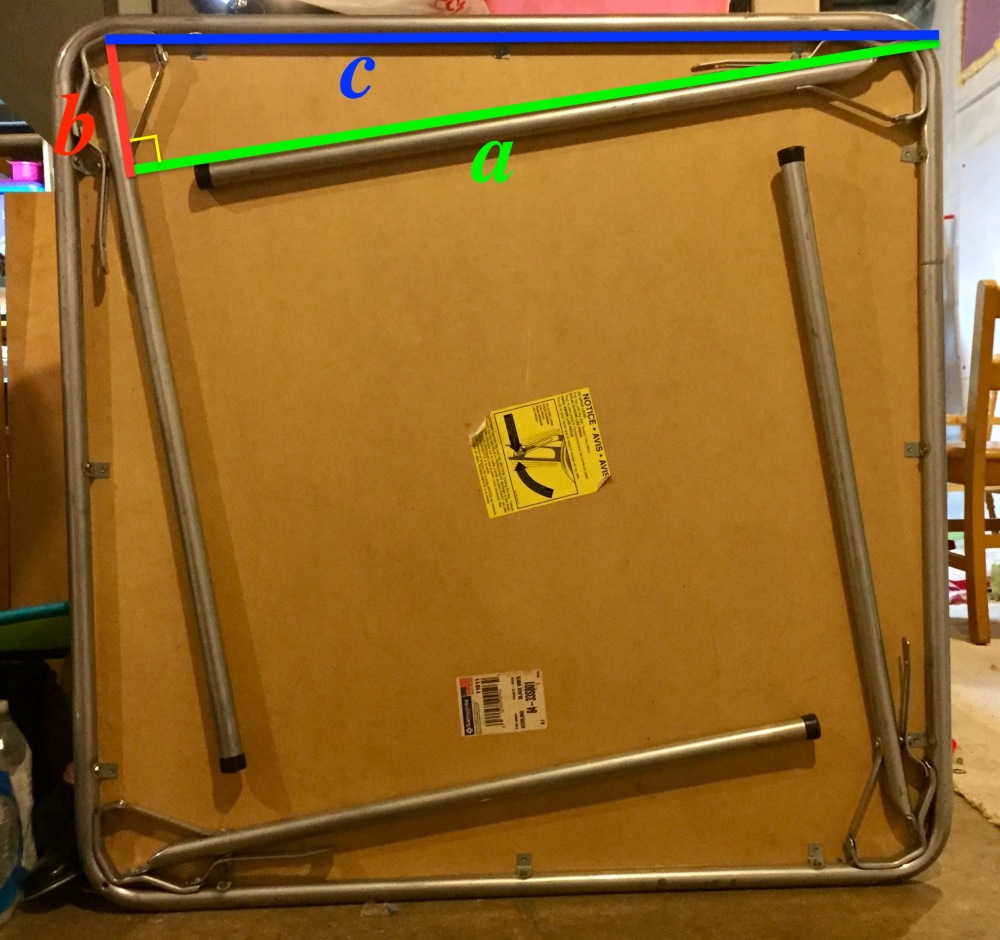
As we see in the photo below, the edge of the table and two adjacent legs form a right triangle with legs a and b and hypotenuse c. The table is square, so it has area c^2. But, the table is divided up into four right triangles each having area \tfrac12 ab and a smaller square of area (a-b)^2. Thus,

c^2=4(\tfrac12 ab)+(a-b)^2=a^2+b^2.

Ta da!

FullSizeRender-3

After I posted this on Twitter I found out that Debra Borkovitz made the same observation about her card table.

4 Comments

  1. Japheth Wood says:
    May 31, 2017 at 3:35 am

    Excellent presentation!
    Here’s another, as an MAA Found Math in 2012.
    http ://www.maa.org/community/columns/maa-found-math/maa-found-math-2012-week-36

  2. Dave Richeson says:
    May 31, 2017 at 8:54 am

    Thanks! I thought about the “found math” site when I took the picture.

  3. J. Franklin Richeson, M.D. says:
    May 31, 2017 at 9:40 am

    Pretty neat!

    >

  4. Pingback: Pythagorean Theorem Lesson – Rethinking Math Class

Comments are closed.